73
Asymmetric waveforms, such as pulse trains, contain DC components that are filtered out
by AC-coupled true RMS measurements.
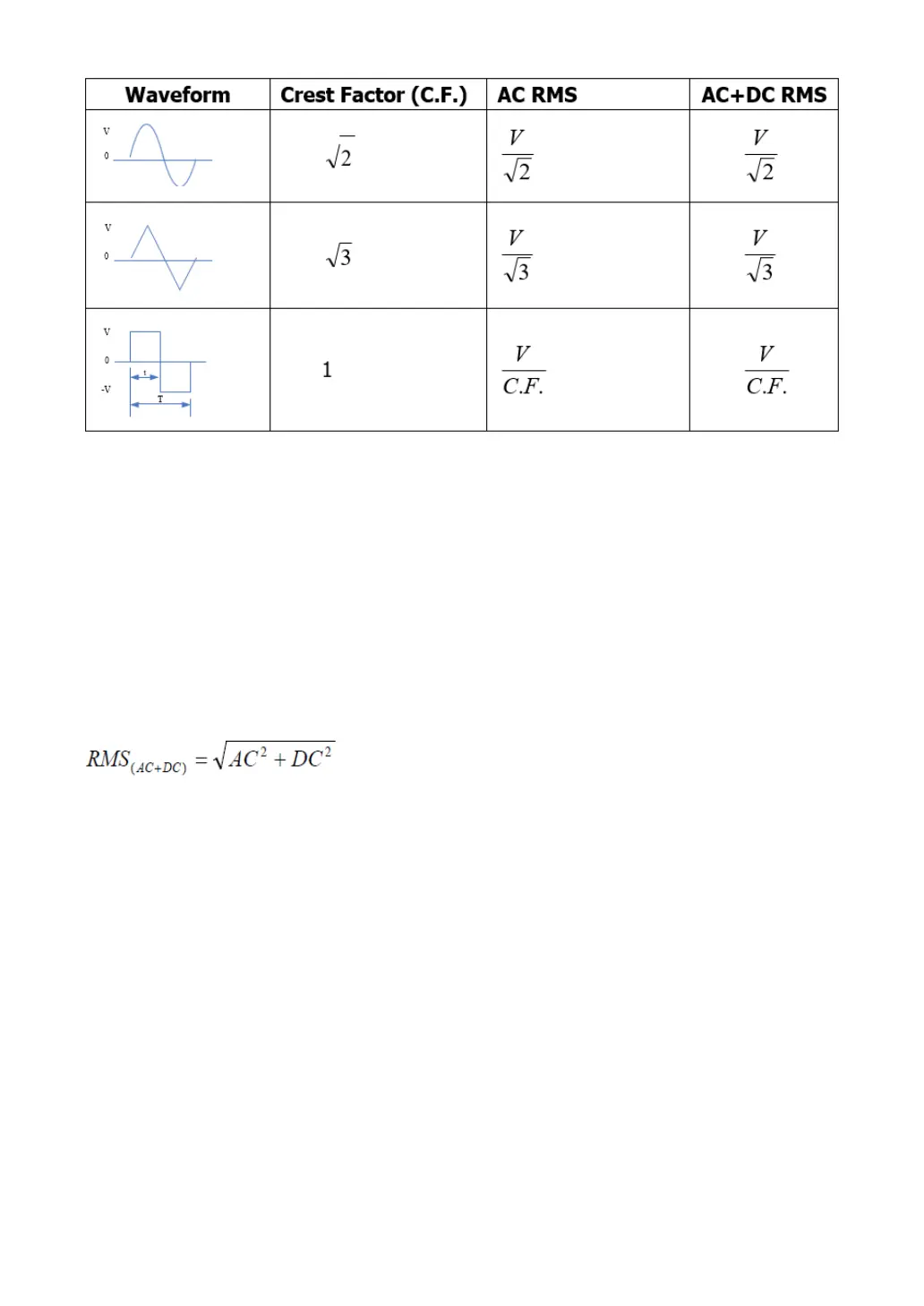
AC coupled true RMS measurements are ideal for measuring small AC signals with DC
offset, such as AC ripple measurements in DC power supply output. However, in some
cases it is necessary to measure AC+DC RMS values. At this point, the DC and AC
components of the signal can be measured respectively using the DC and AC voltage
functions, and then the AC+DC RMS can be calculated according to the following formula.
DC voltage measurements need to use 6.5 bit accuracy to achieve the best AC
suppression.
True RMS accuracy and high frequency signal components
A common misconception is that because the AC multimeter measures true RMS, its sine
wave accuracy index applies to all waveforms. In fact, the waveform of the input signal can
have a significant impact on the measurement accuracy of any multimeter, especially
when the input signal contains high-frequency instrument bandwidth.
For example, consider the waveform that is most challenging for a multimeter, the pulse
train. The pulse width of the waveform largely determines the high frequency component.
The spectrum of a single pulse is determined by its Fourier integral. The spectrum of the
pulse train is a Fourier series sampled along the Fourier integral at a frequency multiple of
the input pulse repetition frequency (PRF).
The following figure shows the Fourier integral of two very different pulses: one has a wide

 Loading...
Loading...