25
16
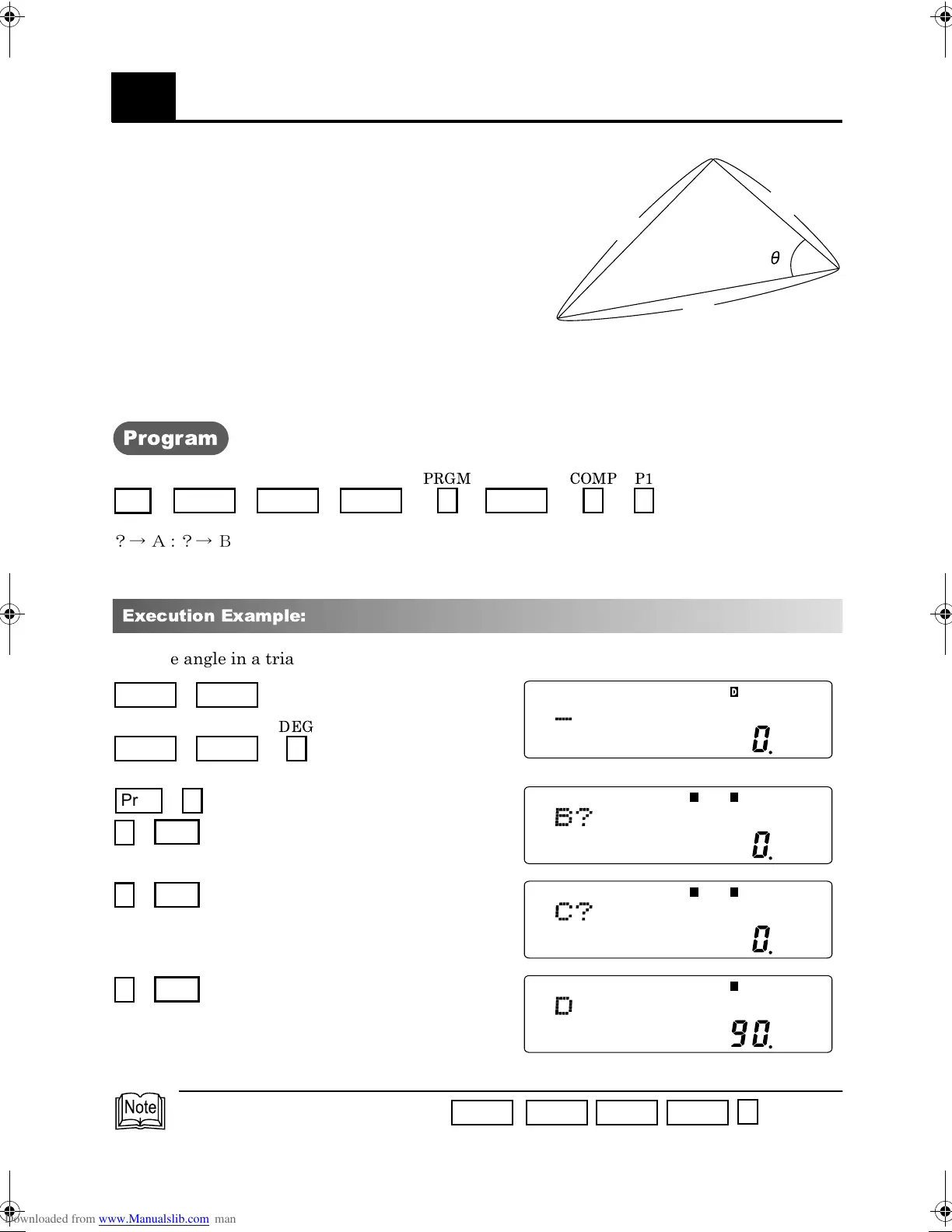
Finding the angles in a triangle
Law of cosines:The relationship between the length
of the sides of a triangle and the angle between is
given by:
Solving this for we obtain the angle in terms of the
lengths:
Program
? → A:? → B:? → C:C O S
-1
((B
2
+C
2
-A
2
)÷2 BC)→ D:D < 33 STEP >
Execution Example:
Find the angle in a triangle with sides of length 3, 4 and 5.
To obtain the answer in radians press before
executing the program.
a
2
b
2
c
2
2bc
θ
cos–+=
a
b
c
θ
θ
1–
cos
b
2
c
2
a
2
–+
2bc
-----------------------------=
ON
MODE MODE MODE
1
PRGM
MODE
1
COMP
1
P1
MODE MODE
MODE MODE
1
DEG
S A
D R
P1
P2 P3 P4
G
Prog
1
5
EXE
S A
D R
P1
P2 P3 P4
G
4
EXE
S A
D R
P1
P2 P3 P4
G
3
EXE
S A
D R
P1
P2 P3 P4
G
8
MODE MODE MODE MODE
2
関数電卓事例集 .book 25 ページ 2002年9月2日 月曜日 午後6時51分

 Loading...
Loading...