Section 5: Data & Graphs Name
Date
Fundamental Topics in Science © 2001 Texas Instruments
Try-It!™ 5-5
Analyze the result quantitatively
There is a statistical variable,
r
2
or
R
2
, called the coefficient of determination, that is often
used to describe numerically how good a fit is. The TI
83 Plus automatically calculates it (and
other statistical variables) when you fit a curve to data. In general terms,
r
2
or
R
2
is calculated by
analyzing how far away each point is from its predicted value on the curve.
The coefficient of determination is
r
2
for
LIN REG
,
EXP REG
,
LN REG
,
PWR REG
, and
R
2
for
QUAD REG
,
CUBIC REG
, and
QUART REG
. It is not calculated for
MED-MED
, or
LOGISTIC
. The
closer that
r
2
or
R
2
is to 1, the better.
Note
: The Fundamental Topics in Science application resets the statistical variables. In order to complete this
exercise, you must run the Science Tools application by selecting it from the APPS menu on the TI
83 Plus instead
of selecting it from the
SCIENCE CHAPTERS
menu in
FUNDAMENTAL TOPICS
.
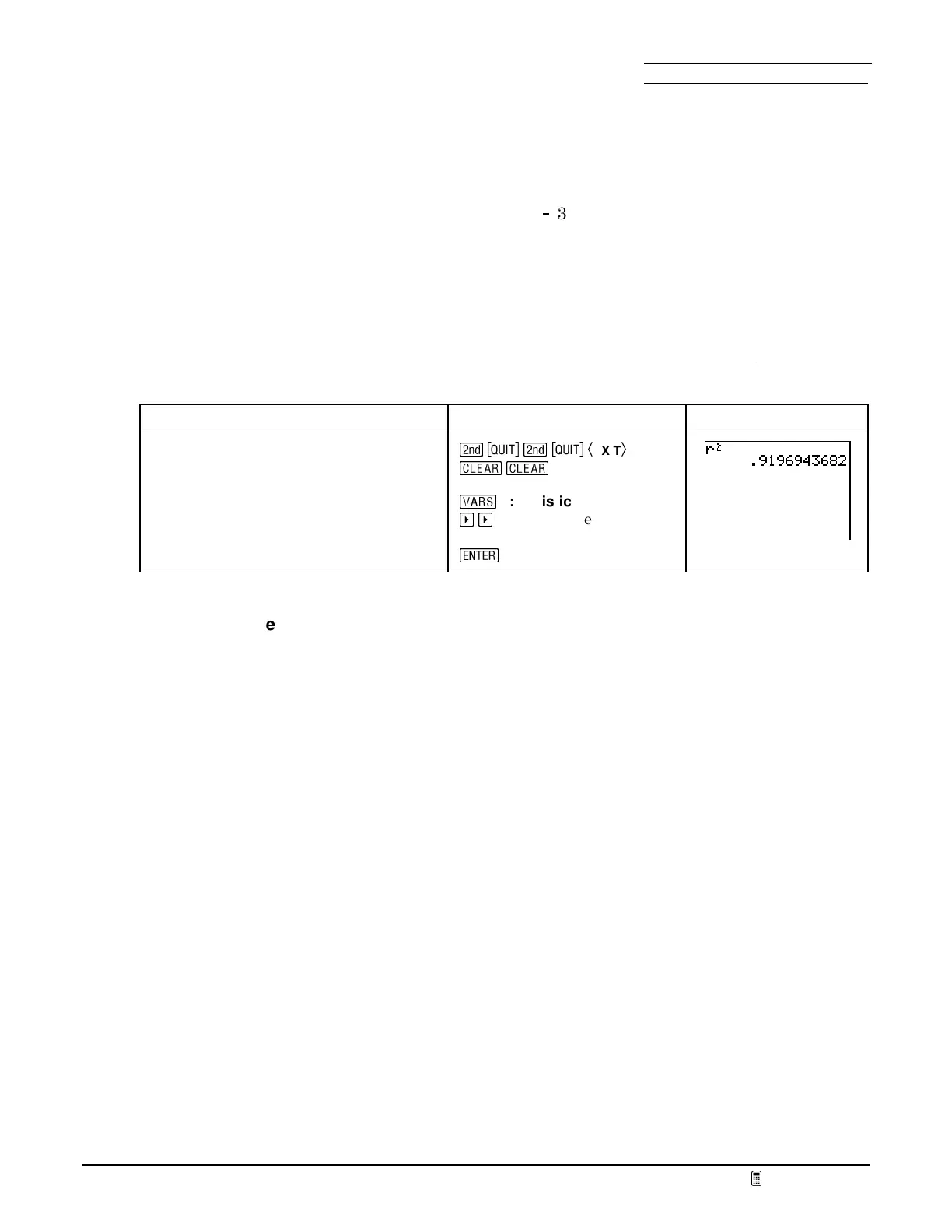
To Do This Press Display
1. Exit
SCIENCE TOOLS
. Clear the home
screen.
2. Copy the
r
2
variable from the
VARS EQ
menu to the home screen
and evaluate it.
\
\
Ã
EXIT
Ä
s
s
r
5:Statistics...
a
a
(to the
EQ
menu)
8:r
2
¯
Fit an exponential curve to the data and analyze the result
.920 is not a particularly good
r
2
. Look for a better fit. Repeat the steps above for an exponential
regression.
Describe how well the exponential function fits the points. What is the
r
2
? How well do you think
the exponential model would predict population in 200 years? Describe the living conditions in
the United States if the exponential model were correct.
#
A mathematical model should be interpreted in relationship to the real world. This example is a
special class of data modeling known as a growth model. While an exponential function may
describe the data fairly well in the data collection period and interpolate well for years in
between measurements, it often is too steep near the end of the data. The logistic model usually
predicts future growth better than linear and exponential models, since the curve levels off after
the “growth spurt.” It is reasonable to expect population will approach a limit rather than
increasing without bound.

 Loading...
Loading...











