TI-83, TI-83 Plus, TI-84 Plus Guide
NOTE: Remember that 2ND ENTER (ENTRY) recalls previously-entered statements so
you do not have to spend time re-entering them.
9.4 Compensating for Change
As you have just seen, the TI-83 closely estimates numerical values of partial derivatives using
its
nDeriv function. This technique can also be very beneficial and help you eliminate many
potential calculation mistakes when you find the rate of change of one input variable with
respect to another input variable (that is, the slope of the tangent line) at a point on a contour
curve.
9.4.1 EVALUATING PARTIAL DERIVATIVES OF MULTIVARIABLE FUNCTIONS The
last few sections of this Guide indicate how to estimate and evaluate partial derivatives using
cross-sectional models. The calculator evaluates partial derivatives calculated directly from
multivariable function formulas using the same procedures. The most important thing to
remember is that you must supply the name of the input variable that is changing and the
values at which the partial derivative is evaluated. We illustrate using the body-mass index
function that is in Example 1 of Section 9.4 of Calculus Concepts:
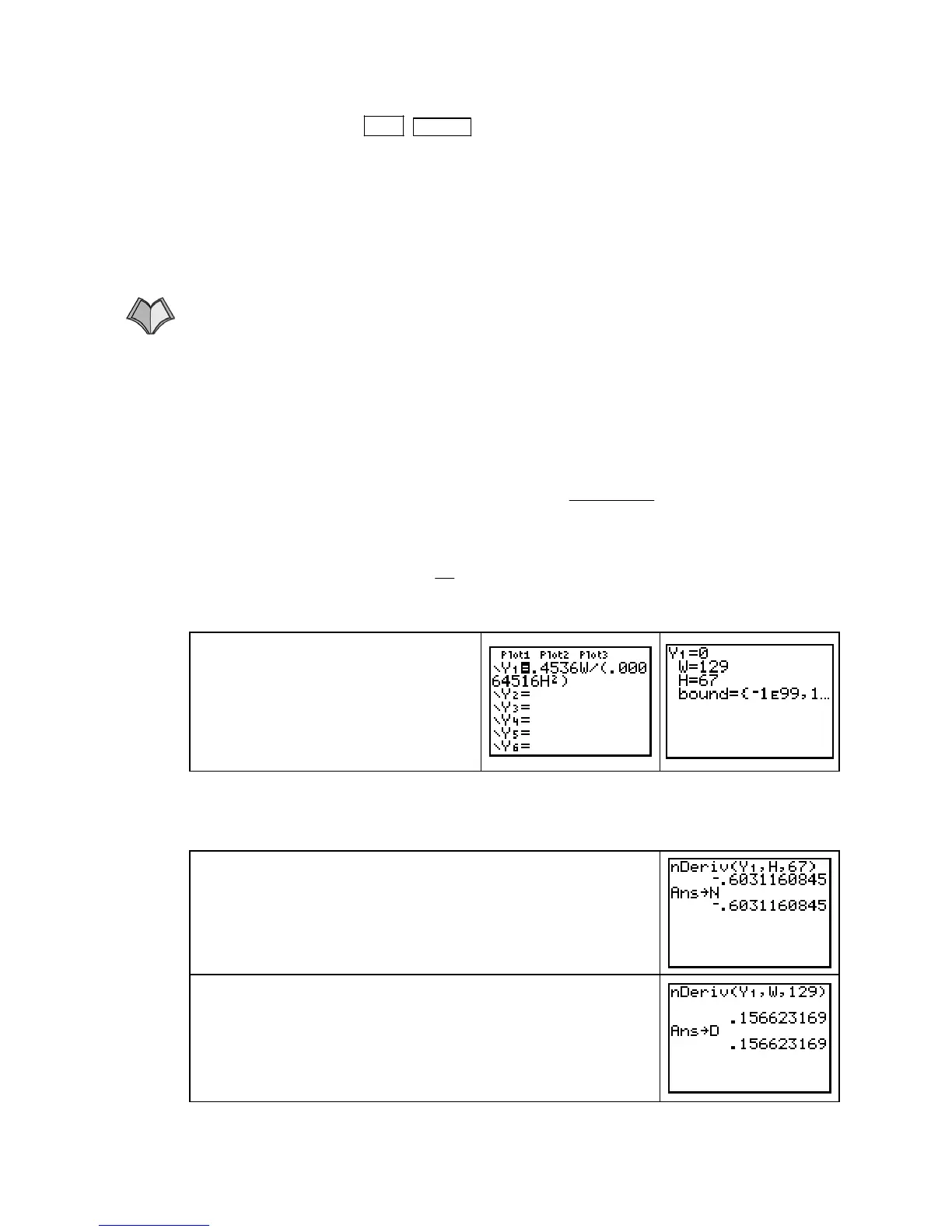
A person’s body-mass index is given by B(h, w) =
0 4536
0 00064516
2
.
.
w
h
where h is the person’s
height in inches and w is the person’s weight in pounds. We first find B
h
and B
w
at a
specific height and weight and then use those values in the next section of this Guide to
find the value of the derivative
dw
dh
at that particular height and weight. The person in
this example is 5 feet 7 inches tall and weighs 129 pounds.
Enter B in the Y1 location of the Y=
list, using the letters
H and W for the
input variables.
Next, use the MATH SOLVER to
store the values of
H and W at the
given point.
CAUTION: The most common mistake made in using this method is forgetting to store the
values of the point at which the derivative is to be evaluated. The
nDeriv instruction specifies
the value at only one of the inputs, and you must tell the calculator the values of all inputs.
Find the value of B
h
at h = 67 and w = 129 by evaluating the ex-
pression shown to the right. The symbol B
h
(or ∂B/∂h) tells you
that h is varying and w is constant, so h is the variable you type
in the numerical derivative.
Next, enter the value of h.
Store this result in
N for use in the next section of this Guide.
Find the value of B
w
at h = 67 and w = 129 by evaluating the ex-
pression shown to the right. The symbol B
w
(or ∂B/∂w) tells you
that w is varying and h is constant, so w is the variable you type
in the numerical derivative.
Next, enter the value of w.
Store this result in
D for use in the next section of this Guide.
Copyright © Houghton Mifflin Company. All rights reserved.
103

 Loading...
Loading...

















