HP 39gs Master Guide

T
T
h
h
e
e
‘
‘
P
P
o
o
l
l
y
y
n
n
o
o
m
m
i
i
a
a
l
l
’
’
g
g
r
r
o
o
u
u
p
p
o
o
f
f
f
f
u
u
n
n
c
c
t
t
i
i
o
o
n
n
s
s
This group of functions is provided to manipulate polynomials.
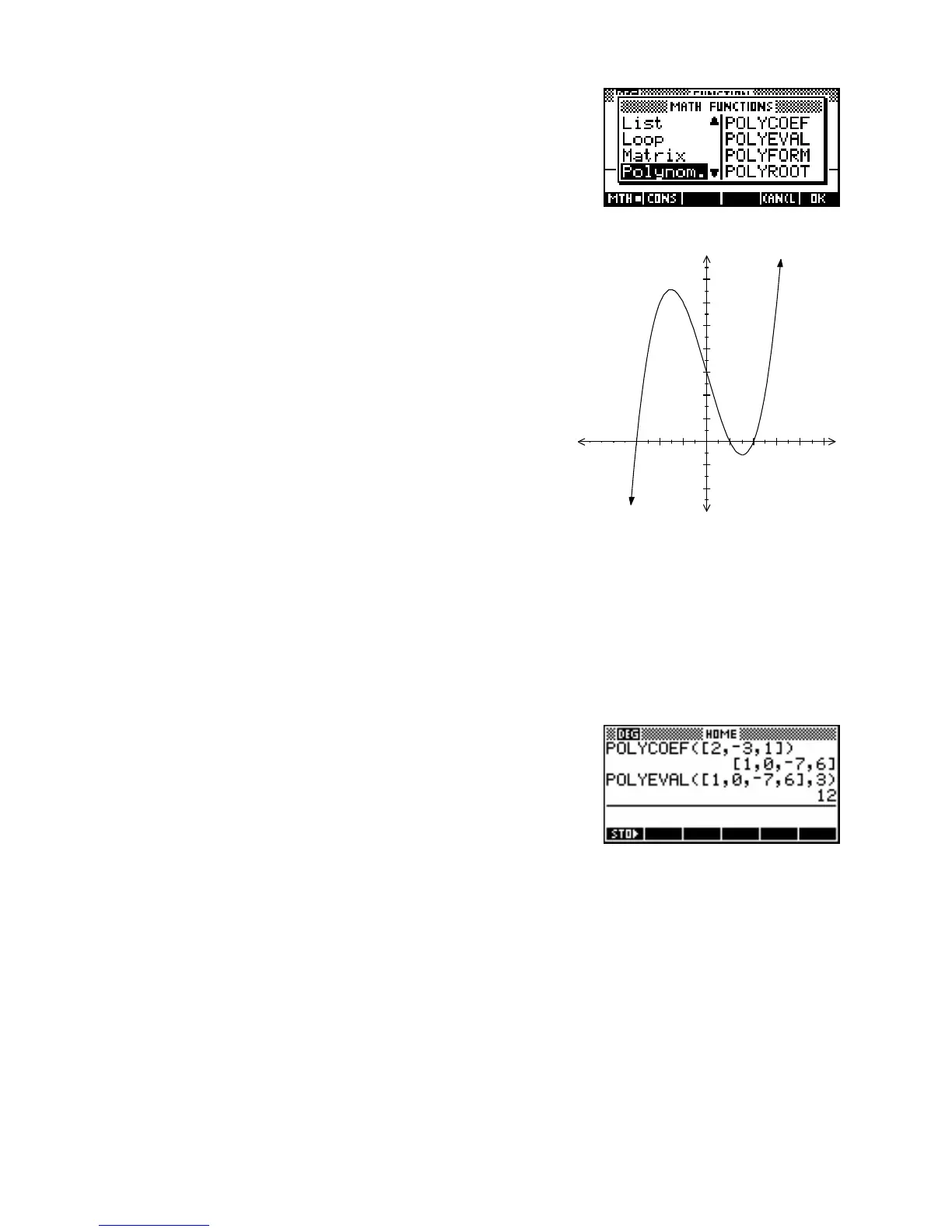
We will use the function shown right to illustrate some of the tools in
the Polynomial group. Its equation is:
() = − 2)(x + 3)(x −1) = − + 6fx (x x
3
7x
f(x)
x
1
2
3
4
5
-1
-2
-3
2
4
6
8
10
12
14
-2
-4
-5 -4
POLYCOEF([root1,root2,…])
1
,,
This function returns the coefficients of a polynomial with roots
xx x
3
,... The roots must be supplied in
2
vector form means in square brackets.
The function
fx above has roots 2, -3 and 1. The screen shot below shows POLYCOEF correctly giving()
the coefficients as 1, 0, -7 and 6 for a final polynomial of
fx 7x()
=
x
3
− + 6 .
POLYEVAL([coeff1,coeff2,…],value)
This function evaluates a polynomial with specified coefficients at the
point specified. The coefficients must be in square brackets, followed by
()
x
3
7 x
the value of x (not in brackets). ie.
fx= − + 6
has value 12
at x = 3.
Note: This is a function that is aimed more at programmers. For normal users it is probably more efficient to
enter the function into the
SYMB view of the Function aplet and then either use the NUM view to find
the function values required, or simply type
F1(3), F1(-2) etc. in the HOME view.
202

HP 39gs Specifications

General

Type	Scientific
Form factor	Pocket
Product color	Gray
Internal memory	0.256 MB

Weight and Dimensions

Weight	249 g
Dimensions (WxDxH)	187 x 94 x 31 mm